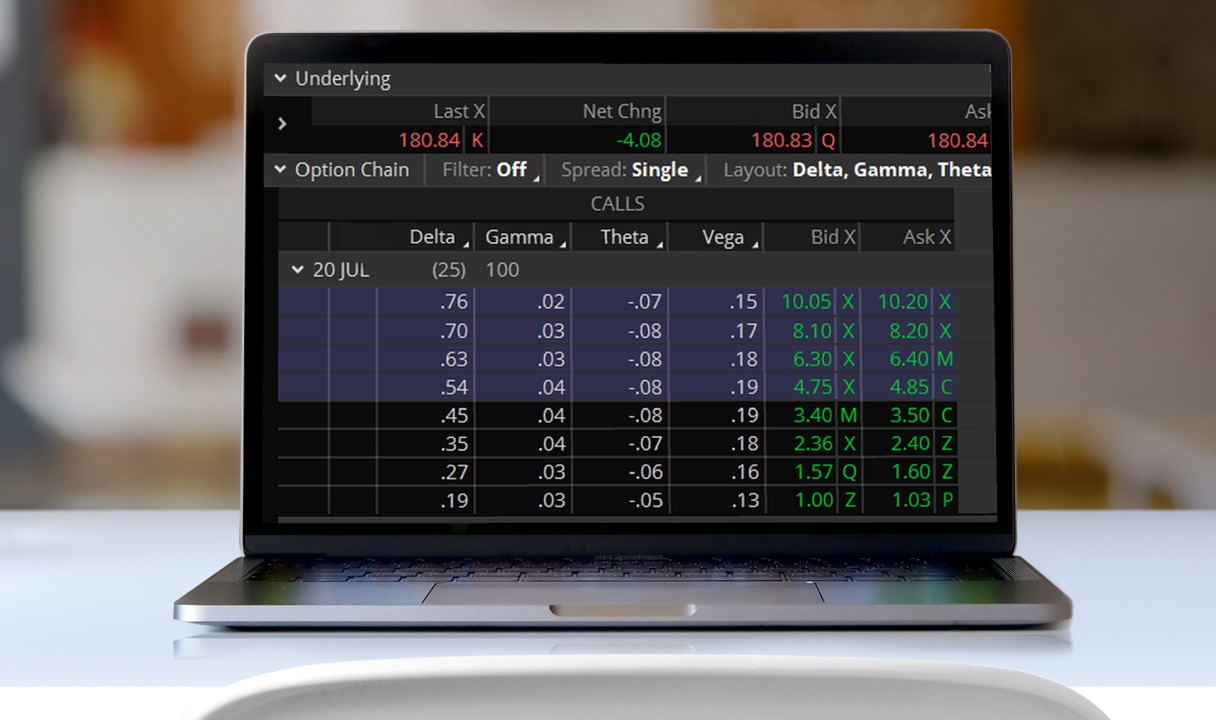
Options Greeks

Upbeat music plays throughout. 
 
Narrator: If you dig deep down into your high school memories, you can probably uncover some facts about Greek mythology.

Like Zeus, the ruler of Olympus, and all the gods. Hades, lord of the underworld. And…all those other guys in between.

Well, those memories aren't so clear anymore. But don't worry. Today, we'll focus on a different group of greeks—the options greeks.

Like the ancient gods, these greeks oversee certain domains, including price, time, and implied volatility. The greeks are an important part of options trading, as they tell you how changes in certain factors may impact the price of an option. So, let's get to know them.

We'll start with delta. Like Zeus, delta is the ruler over all the other options greeks because it often has the biggest impact on the value of an option.

Delta's domain is price—it identifies how much the options premium may change if the underlying price changes $1.

This means that a call option with a delta of .40 would be expected to increase by $0.40 if the underlying rose $1.

Delta has another important use as well. Some traders might use it to estimate the probability of an option expiring in the money. For example, an option with a delta of .40 can also be interpreted as having a 40% chance of expiring in the money. The lower the delta, the lower the odds that the option will expire in the money.

One important thing to note about delta is that it doesn't have a constant rate of change. It grows as an option moves further in the money and shrinks as it moves further out of the money. To understand how this works, let's look at the next greek: gamma.

Gamma is delta's Hermes, his right-hand man in the price domain. Gamma measures delta's expected rate of change.

If an option has a delta of .40 and a gamma of .05, the premium would be expected to change $0.40 with the first $1 move in the underlying. Then, to figure out the impact of the next dollar move, simply add delta and gamma together to find the new delta: .45.

Let's move on to theta, the greek of time decay. Theta estimates how much value slips away from an option with each passing day.

If an option has a theta of negative .04, it would be expected to lose $0.04 of value every day.

Remember, time decay works against buyers and for sellers.

Finally, there's vega, whose domain is implied volatility. Vega estimates how much the premium may change with each one percentage point change in implied volatility.

There are a lot of factors that could cause a spike in implied volatility: earnings announcements, political conditions, and even weather. Depending on the strategy you choose, a spike in volatility could be a blessing, a curse, or have a very small impact. And the further out an options expiration is, the higher its vega will be. In other words, options with a longer expiration may react more to a change in volatility.

If an option has a vega of .03 and implied volatility decreases one percentage point, the premium would be expected to drop $0.03.

Now, let's talk about the little brother of the options greeks: rho. Rho identifies how much an options premium may move if interest rates change. Because rates change slowly, they have a smaller impact on options trading.

Like all little siblings, though, rho is often left out of discussions about the greeks. Nonetheless, rho is still part of the family, so he's still worth mentioning here. Now, let's pull our greek council together and look at how they can be used to analyze the sensitivities of a single option. To set the stage, let's say your options premium is $1.30. And your option has a delta of .35, gamma of .06, theta of .02, and vega of .07.

Today, price moves from $45 to $46, and the premium increases $0.35 to $1.65. Because a day has passed, the premium decreases $0.02 due to theta.

Tomorrow, price moves from $46 to $47. The premium increases $0.41 to $2.04; this is delta plus gamma.

Also, another day gone by, means another day of time decay, and another $0.02 down the drain.

Implied volatility rises one percentage point, increasing the premium by $0.07 to $2.09.

Putting all these factors together shows how a relatively small change in the underlying can lead to a pretty significant change in the options premium.

The options greeks are a helpful crew to know. They help you understand the impact various factors can have on options trades. You'll get to know them very well as you continue your options education.

On-screen text: [Schwab logo] Own your tomorrow^®